Based on a course by Federica Sbergami^[1]^[2]^[3]

Languages

Microeconomics Principles and Concept ● Supply and demand: How markets work ● Elasticity and its application ● Supply, demand and government policies ● Consumer and producer surplus ● Externalities and the role of government ● Principles and Dilemmas of Public Goods in the Market Economy ● The costs of production ● Firms in competitive markets ● Monopoly ● Oligopoly ● Monopolisitc competition

Welfare economics is an important branch of economics that focuses on understanding and evaluating the efficiency and equity of resource allocation by the market. This discipline seeks to determine whether the allocation of resources through the market mechanism maximises collective well-being. It operates through two distinct but complementary prisms of analysis: positive analysis and normative analysis.

Positive analysis seeks to observe and describe economic phenomena objectively. For example, it may look at the effects of a change in tax policy on incomes without judging whether these effects are desirable or not. Normative analysis, on the other hand, ventures into the realm of value judgements, asking what ought to be. It assesses, for example, whether the allocation of resources by the market is fair or efficient, going beyond simple observation to question the desirability of economic outcomes. In welfare economics, tools such as consumer and producer surplus are used to measure the benefits that individuals and firms derive from participating in the market. These tools help to assess whether the market allocates resources in a way that maximises collective welfare, which is the sum of the individual benefits of all market participants.

Welfare economics is also concerned with questions of equity and efficiency. For example, it may examine whether the distribution of resources and wealth is equitable, or whether the market manages to allocate resources in such a way as to maximise production and the satisfaction of society's needs and desires. It also looks at phenomena such as externalities and public goods, where market forces may not lead to an efficient allocation of resources. Externalities, such as pollution, where the costs or benefits of an economic activity affect others who are not directly involved in the transaction, are a classic example of a market failure that welfare economics seeks to understand and correct. The application of welfare economics in real life is vast. For example, governments use its principles to design tax policies that not only generate revenue, but also seek to spread the tax burden fairly. Similarly, in the case of environmental regulation, welfare economics helps to balance the economic costs of reducing pollution with the benefits in terms of public health and the environment.

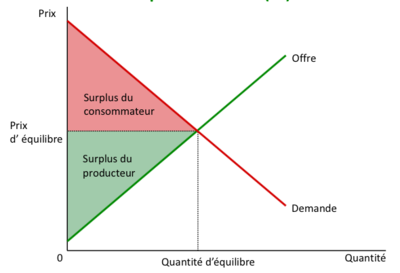
To assess the benefits derived by consumers and producers from their participation in the market, welfare economics relies on the concepts of consumer and producer surplus. These concepts are fundamental to understanding how the market allocates resources and to assessing whether this allocation maximises society's overall well-being. Consumer surplus is the measure of the benefits that consumers obtain by purchasing goods and services. More precisely, it represents the difference between what consumers are prepared to pay for a good or service and what they actually pay. If, for example, a consumer is prepared to pay 15 euros for a product but only pays 10 euros for it, their surplus is 5 euros. This surplus reflects the benefit or satisfaction obtained over and above the cost incurred. On the other hand, the producer surplus is the difference between the amount producers receive for selling their goods or services and the cost of producing them. It is essentially the profit that producers make from selling their products over and above their production costs. For example, if a producer sells a good for €20 when the cost of production is €15, his surplus is €5.

In a perfectly functioning market, with no flaws (such as externalities, public goods, imperfect information or monopolies), the market's allocation of resources is said to be "efficient" in the Pareto sense. This means that no one can be made better off without making someone else worse off. In such an ideal scenario, the market succeeds in maximising aggregate welfare, which is the sum of consumer surplus and producer surplus. This translates into an allocation of resources that not only maximises aggregate output, but does so in a way where the benefits of some are not obtained at the expense of others. This idealised analysis of the perfect market serves as a benchmark for assessing the performance of real markets. Economists can then identify market failures and propose policy interventions to correct these failures, with the aim of improving the efficiency and equity of resource allocation.

Economic Surplus Analysis[modifier | modifier le wikicode]

Consumer and producer surplus are fundamental concepts in economics that allow us to analyse and evaluate the efficiency with which markets allocate resources. These two measures help us to understand the benefits that consumers and producers derive from their interactions in the market. They are essential indicators for assessing market performance and for guiding economic policies aimed at improving the efficiency and equity of resource allocation.

Consumer surplus is the difference between what consumers are willing to pay for a good or service and what they actually pay. It therefore represents the benefit or advantage that a consumer obtains by acquiring a good at a lower price than he was prepared to pay. For example, if an individual is prepared to pay 15 euros for a book but only pays 10 euros for it, their consumer surplus is 5 euros. This surplus indicates the additional value that the consumer perceives in the purchase over and above the price paid. On the other hand, the producer surplus is the difference between the price at which a good is sold and the minimum cost for which the producer was prepared to sell it. In other words, it is the profit or advantage that a producer derives from the sale of a good, over and above its production costs. Let's take the example of a farmer who sells apples: if the production cost of an apple is €0.50 and he sells it for €1, his producer surplus per apple is €0.50. This surplus reflects the economic gain made by the producer on the sale.

In an ideally functioning market, where there are no market failures such as externalities or monopolies, total surplus (the sum of consumer and producer surplus) is maximised. This means that resources are allocated as efficiently as possible, thereby maximising general welfare. For example, in a competitive fruit and vegetable market with no market failures, the equilibrium price and quantity result in maximum consumer and producer surplus, reflecting an efficient allocation of agricultural resources. However, in reality, markets can often be imperfect due to various failures. For example, in the case of industrial pollution (a negative externality), the cost of pollution is not factored into the price of the product, which can lead to overproduction and overconsumption of that product, thereby reducing social welfare. Government interventions, such as taxing polluters or environmental regulations, aim to correct these shortcomings and bring resource allocation closer to ideal efficiency.

Understanding Market Demand[modifier | modifier le wikicode]

Consumer surplus is an economic concept that measures the benefit or advantage that a consumer derives from participating in the market. This surplus is calculated as the difference between the price the consumer is prepared to pay for a good or service (his reserve price) and the price he pays to acquire it. To illustrate this concept, let's take the example of a consumer who is considering buying a smartphone. If this consumer is prepared to pay up to 800 euros for a particular smartphone, but finds an offer at 600 euros, his consumer surplus is 200 euros. This means that he obtains an additional benefit of 200 euros in terms of satisfaction or perceived value, because he has paid much less for the smartphone than the maximum price he was prepared to pay.

This consumer surplus is a way of quantifying the welfare gain that consumers obtain by participating in the market. It represents the difference between their subjective assessment of the value of a good and the amount they actually spend to obtain it. In a market economy, consumer surplus is often used to assess the efficiency of resource allocation and to analyse the impact of economic policies, such as taxes or subsidies, on consumer welfare.

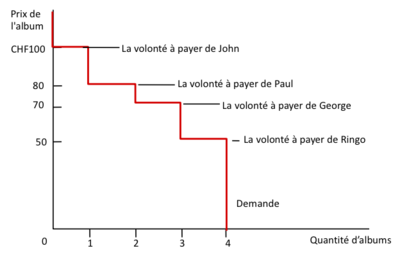
This table is divided into two main sections: the first lists the willingness to pay of different buyers for original Rolling Stones albums, and the second shows how the quantity demanded varies with the price of the albums.

In the first section, we have four buyers - John, Paul, George, and Ringo - each with a different willingness to pay for an album. John is willing to pay the most, up to CHF 100 (Swiss francs), while Ringo is willing to pay the least, with a maximum of CHF 50. The second section of the table details how the price affects the quantity requested. When the price is over CHF 100, none of the buyers is prepared to buy the album, which means that the quantity requested is zero. If the price is between CHF 80 and CHF 100, only John is interested, as he is the only one willing to pay in this price range, so the quantity requested is one album. If the price falls to between CHF 70 and CHF 80, both John and Paul will be willing to buy the album, increasing the quantity requested to two. Between CHF 50 and CHF 70, three buyers (John, Paul and George) are prepared to buy, and finally, if the price is less than or equal to CHF 50, all the buyers are prepared to buy, increasing the quantity demanded to four albums.

Let's now analyse the consumer surplus for each price. If the albums are sold at CHF 50, John, Paul and George all have consumer surplus, which is the difference between their willingness to pay and the selling price. For example, if John buys at CHF 50, his surplus is CHF 100 - CHF 50 = CHF 50. Similarly, Paul would have a surplus of CHF 30 and George CHF 20. Ringo would have no surplus because his reserve price is equal to the market price. This table is a good illustration of the law of demand, which states that the quantity demanded of a good increases as its price falls, provided that all other factors remain constant. It also shows how consumer surplus varies for each individual as a function of the price of the good.

In the context of pricing policy, if a seller wanted to maximise revenue without regard to consumer surplus, he could set the price at CHF 70, thus selling two albums to John and Paul, which is less than the maximum quantity but at a higher price than if all the albums were sold at CHF 50. However, to maximise total welfare (the sum of consumer and producer surpluses), the seller would have to strike a balance between setting the price high enough to cover costs and generate a profit, while keeping it low enough to allow as many buyers as possible to benefit from a significant surplus.

Construction of the Aggregate Demand Curve[modifier | modifier le wikicode]

The aggregate demand curve represents the total quantity of a certain good or service that all consumers in a market are willing to buy at each possible price level. It is constructed by adding together the quantities demanded by all consumers at each price level. The curve shows the relationship between the price of a good and the quantity of that good that consumers are willing to buy, assuming that all other factors affecting demand remain constant (ceteris paribus).

In general, the aggregate demand curve has a negative slope, reflecting the law of demand: when the price of a good increases, the quantity demanded of that good decreases, and vice versa. This can be explained by two main effects:

The substitution effect: When the price of a good increases, consumers will look for cheaper substitutes, thus reducing the quantity demanded of the more expensive good;
The income effect: An increase in price reduces consumers' purchasing power, which reduces their ability to buy products at the same level as before.

In practice, the demand curve can be affected by many factors other than price, such as changes in consumer incomes, changes in tastes and preferences, changes in the prices of substitute and complementary goods, and future consumer expectations. When these factors change, they can shift the demand curve to the left or right.

To construct the aggregate demand curve from the data in the table provided, we would add up the quantity that each buyer is prepared to purchase at different price levels. Then, by placing the price on the vertical axis and the quantity on the horizontal axis, we would draw a curve linking the different points corresponding to the quantities demanded accumulated at each price. This aggregate demand curve would then be used to analyse how price changes influence the total quantity demanded on the market.

This image shows an aggregate demand curve for albums, probably in a hypothetical or case study context. This curve is plotted on a graph with the price of the album on the vertical axis (CHF) and the quantity of albums on the horizontal axis.

The curve is made up of horizontal segments at prices that correspond to the willingness of individual buyers to pay for the album:

John is willing to pay up to 100 CHF, which is the highest price on the demand curve.
Paul has a willingness to pay of up to 80 CHF.
George is willing to pay up to 70 CHF.
Ringo has the lowest willingness to pay, at 50 CHF.

The "stair step" formed by the curve indicates that each buyer has a specific willingness to pay and that no buyer is prepared to pay more than his or her indicated willingness to pay. When the price is above the willingness to pay of all buyers, the quantity demanded is zero. As the price decreases to match the willingness to pay of each successive buyer, the quantity demanded increases in stages. The curve clearly shows the law of demand: as the price falls, the quantity demanded increases. At a price of CHF 100, no albums are in demand. When the price drops to CHF 80, John starts asking for an album, which increases the quantity requested to 1. At CHF 70, Paul joins John, bringing demand to 2 albums. At CHF 50, all the buyers are ready to buy the album, bringing total demand to 4.

This graph also illustrates the concept of consumer surplus. For example, if the albums are sold at CHF 50 each, John benefits from a consumer surplus equal to the difference between his willingness to pay (CHF 100) and the price of the album (CHF 50), i.e. a surplus of CHF 50. Similar calculations can be made for Paul and George.

In a real-world context, this representation would help sellers understand how price influences demand and could be used to determine the optimal selling price that maximises either quantity sold or total revenue, depending on the seller's business objective. However, it should be noted that in real market scenarios, consumer preferences are not always so clearly defined and can be influenced by a multitude of factors other than price alone.

Valuation of Consumer Surplus[modifier | modifier le wikicode]

Consumer surplus is an economic concept that captures the difference between what consumers are prepared to pay for a good or service and what they actually pay. This surplus represents the advantage or benefit that consumers derive from buying a good at a price lower than their reserve price, i.e. the maximum they would be prepared to pay. To illustrate this concept, imagine that a consumer is considering buying a new pair of shoes. If this consumer is prepared to pay up to 120 euros for these shoes, but finds them on sale for 80 euros, his consumer surplus is 40 euros. This calculation is based on the idea that the consumer has made a "saving" of 40 euros compared with what he was prepared to pay initially, which gives him a welfare gain.

Consumer surplus is therefore a measure of the utility gained by consumers when they carry out transactions on markets at prices that are lower than their personal valuations of the goods and services purchased. It is an important concept because it allows us to assess the economic efficiency of markets and to analyse how price changes, due to economic policies or market fluctuations, can influence consumer well-being. When the consumer surplus of all individuals in a market is added together, we obtain a measure of the total welfare that the market generates for consumers. A market is considered to be more efficient if it maximises total consumer surplus, i.e. if consumers together derive the maximum benefit from their purchases relative to what they would have been prepared to spend.

This graph provides a visual illustration of the notion of consumer surplus in a particular market context. On this graph, we see John's willingness to pay for an album, which is indicated by a point on the price axis at CHF 100. The market price is represented by a horizontal line at CHF 80. The difference between John's willingness to pay (CHF 100) and the market price (CHF 80) is represented by a coloured area, which illustrates John's consumer surplus, equivalent to CHF 20. This consumer surplus of CHF 20 indicates the economic advantage that John obtains by buying the album at a lower price than he was prepared to pay. It represents the additional welfare gain or utility that John perceives from making this transaction. In more general terms, consumer surplus is an indicator of the economic benefit obtained by consumers when they purchase goods or services at prices below their reservation prices.

In the context of a market analysis, this surplus can be used to assess how the change in prices would affect consumer welfare. If the market price were to rise, for example, John's consumer surplus would fall, while a fall in the market price would increase his surplus. This could also influence John's decision whether or not to proceed with the purchase, depending on how prices change. The demand curve, shown in the graph, represents the quantity of albums that consumers are prepared to buy at different price levels. It shows the typical inverse relationship between price and quantity demanded: as the price decreases, the quantity demanded increases.

In a real-life situation, understanding consumer surplus can help sellers set their prices strategically to maximise both consumer welfare and their own profit. It can also inform policy makers who are considering measures such as taxes or subsidies, which would directly affect market prices and therefore consumer surplus.

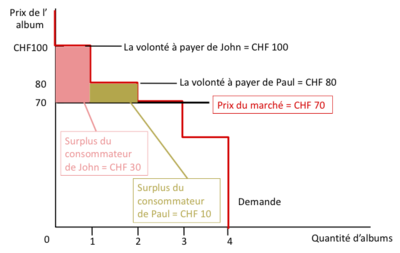
This graph shows the consumer surplus for two individuals, John and Paul, in a hypothetical market where albums are sold. The consumer surplus is visualised by the coloured areas and is calculated as the difference between the individual's willingness to pay (his reserve price) and the current market price.

For John: His willingness to pay is CHF 100. The market price is CHF 70. The difference between these two amounts is CHF 30, which represents John's consumer surplus.
For Paul: His willingness to pay is CHF 80, and with the market price at CHF 70, his consumer surplus is CHF 10.

This graph illustrates that when the market price is lower than the consumers' willingness to pay, each of them obtains a surplus, which is a measure of their gain in terms of economic well-being. John enjoys a greater surplus because the difference between his willingness to pay and the market price is greater.

The interesting aspect here is that consumer surplus increases as the market price falls. If the market price were higher, for example at CHF 80, Paul would have no consumer surplus and John's surplus would be reduced. Conversely, if the price were lower than CHF 70, both consumers would see their surplus increase. This illustration also shows the effect of the elasticity of demand. If the price falls and more consumers like George or Ringo enter the market because of their own willingness to pay, the overall consumer surplus in the market would increase. In reality, understanding consumer surplus can help companies set prices that maximise profits while keeping customers satisfied. In addition, policy makers can use this information to assess the impact of fiscal policies, such as sales taxes, on consumer welfare.

Consumer surplus is the difference between what consumers are prepared to pay for a good or service (reflecting the value they place on that good) and what they pay on the market. Consumer surplus is therefore a monetary measure of the benefit or utility that consumers obtain from the exchange. Graphically, consumer surplus is represented by the area under the demand curve and above the market price level. On a conventional diagram where the demand curve slopes downwards from left to right, this area takes the form of a triangle or a trapezoid, depending on the precise shape of the demand curve.

Let's take a simple example to illustrate: if the demand curve is linear, and the market price is below the maximum price that some consumers are prepared to pay, the consumer surplus is represented by a triangle. The base of the triangle is the difference between the maximum price willing to be paid (the start of the demand curve on the y-axis) and the market price. The height of the triangle is the quantity purchased at the market price. This surplus represents a gain for consumers, as it indicates that they have been able to acquire a good for less than they were prepared to pay for it, and this gain is often interpreted as a measure of their satisfaction or well-being resulting from their participation in the market. In other words, it quantifies the benefit that consumers derive from the operation of the market in terms of satisfaction or utility in relation to the money spent.

This graph shows a classic representation of the demand curve and consumer surplus in an economic context. The demand curve, plotted in red, illustrates the inverse relationship between the price and the quantity demanded of a good or service, meaning that when the price increases, the quantity demanded decreases, and vice versa. This relationship is a fundamental law in economics known as the law of demand.

On this graph, the market price is indicated by a horizontal line that intersects the demand curve at a specific point, reflecting the price level at which the good is currently traded on the market. The point where this horizontal line intersects the quantity axis determines the quantity of goods purchased at that market price. Consumer surplus is represented by the area coloured green. This surplus is the difference between the price consumers are willing to pay and the price they actually pay. It is expressed as the area under the demand curve, but above the market price, up to the quantity purchased. This gap represents the additional benefit or utility that consumers derive from buying the good at a price below their maximum willingness to pay.

In this space, each point on the demand curve represents a maximum price that a consumer is prepared to pay for a given quantity of goods. The highest price that some consumers are prepared to pay is illustrated by the highest point on the demand curve, marked as P1. At this price level, the quantity demanded would be zero, since this is the highest price anyone would be prepared to pay and there would be no buyers at this level. As the price falls to the market price, more consumers are willing to buy the good, which is indicated by the point where the market price line intersects the demand curve at quantity Q1. Consumer surplus is an important measure of the total economic benefit that consumers derive from purchasing goods on a market. It is essential for economic analysis because it allows us to understand how price changes affect not only the quantity of goods traded but also consumer welfare. When the market price falls, consumer surplus increases, as consumers derive greater satisfaction from their ability to buy at a lower price than they were prepared to pay.

In practice, companies can take a close interest in consumer surplus when making decisions about the pricing of their products. A price that is too high could significantly reduce consumer surplus and potentially reduce the quantity demanded. Conversely, a price that is too low could increase the quantity demanded but reduce the company's profit margins. The aim is often to find a balance that maximises profits while maintaining a consumer surplus high enough to keep customers satisfied and loyal.

Impact of Changes on Consumer Surplus[modifier | modifier le wikicode]

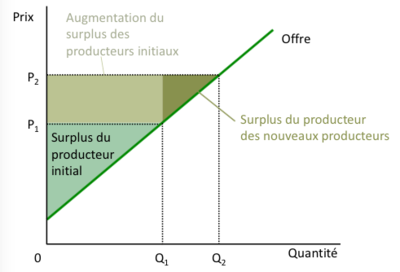
The change in consumer surplus due to a price change is represented by the difference between the areas under the demand curve at the two price levels. When the price of a good or service decreases, consumer surplus increases because consumers benefit from a greater difference between what they are willing to pay and what they do pay. This increase is visualised as the additional area that forms between the demand curve and the new lower price.

Conversely, if the price rises, the consumer surplus falls. This reduction is represented by the loss of the area that existed between the two price levels on the demand curve. Consumer surplus is therefore reduced because consumers are paying a price that is closer to their reserve price, and some consumers who were prepared to buy at the lower price may decide not to buy at the higher price.

This relationship between price change and consumer surplus is fundamental in economics because it reflects the direct impact of price changes on consumer welfare. It is particularly relevant when analysing the impact of economic policies such as product taxation or subsidies, which alter market prices and therefore consumer surplus. Economists and policy makers can use this information to assess the efficiency of markets and the effect of policy changes on general welfare.

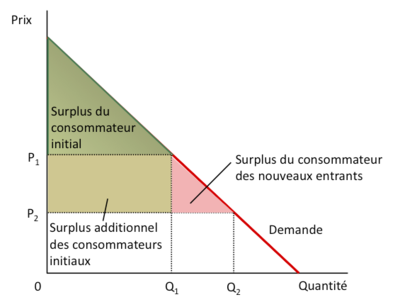
This graph illustrates the changes in consumer surplus that result from a reduction in the price of a good or service on a market. The demand curve, represented by the red line descending from left to right, shows the relationship between the price and the quantity demanded of a good.

Initially, the market price is at P1, where the quantity demanded is Q1. The initial consumer surplus at this price level is represented by the area coloured green, which is the area below the demand curve and above the P1 price line, up to Q1. When the price falls to P2, the consumer surplus expands to include not only the initial green area but also the additional area coloured yellow. This yellow area represents the additional surplus that the original consumers receive as a result of the price reduction; they pay less than they were initially willing to pay for Q1 units of the good. Furthermore, at this new lower price, additional consumers enter the market, increasing the quantity demanded at Q2. The consumer surplus of the new entrants is represented by the area coloured red. This is the area below the demand curve and above the price line P2, between Q1 and Q2. These consumers were not prepared to buy the good at the initial price P1, but are encouraged to make purchases because of the reduced price.

Together, the initial consumer surplus, the additional surplus of the initial consumers, and the consumer surplus of the new entrants represent the total consumer surplus after the price cut. This total surplus is an indication of the total economic benefit or welfare that consumers derive from their participation in the market after the price change.

Analysing the impact of a price change on consumer surplus is essential to understanding the economic implications of pricing policies. For example, price reductions can be used as incentives to increase consumption or to make a product more accessible in a market. Conversely, a price increase could reduce consumer surplus and potentially reduce overall demand for the good. Commenting further, it is important to note that if the price falls even further, initial consumers would benefit from an even greater surplus and the number of new entrants could increase, which would broaden total welfare in the market. However, this drop in price could have consequences for producers, notably a reduction in their surplus (not shown in this graph). This is the kind of analysis an economist might use to assess the effects of a pricing policy or to understand market dynamics after changes in supply or demand.

Fundamentals of Market Supply[modifier | modifier le wikicode]

Producer surplus is an economic concept that reflects the profit made by producers when they sell their goods and services on the market. It is the difference between the income they receive from selling these goods and the cost of producing them. In other words, it is the amount of money that producers earn after covering their production costs.

In practice, producer surplus is represented by the area above the supply curve (which indicates the marginal cost of production or the producers' reserve price) and below the market price at which the goods are sold. If a producer is willing to sell a good for at least €10, but sells it for €15, his producer surplus for that good is €5. This represents the net gain above what was the minimum acceptable for the producer.

Producer surplus is an indicator of the economic health of companies and the profitability of markets. A high surplus may indicate a beneficial market for producers, where they are able to sell at prices substantially higher than their costs. This can stimulate investment, production expansion and innovation. However, it is important to note that producer surplus can be affected by many factors, including changes in production technology, input costs, market competition, and government policies such as taxes and subsidies. A thorough understanding of producer surplus can help policy makers and companies make informed decisions that affect production, pricing and overall market strategy.

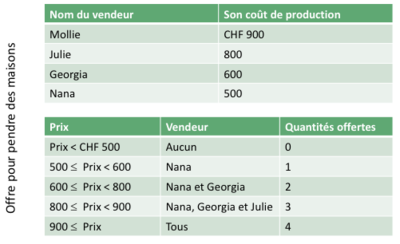
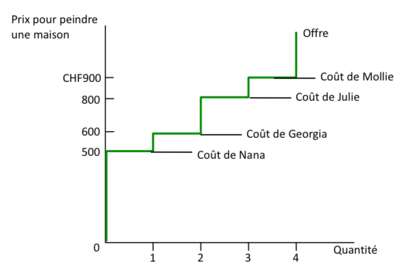
This table provides information on the production costs of different sellers and how these costs influence the quantity of goods they are prepared to offer at different price levels on the market for a specific product, in this case houses.

In the first part of the table, four sellers - Mollie, Julie, Georgia and Nana - are listed with their respective production costs for hanging houses. These costs range from CHF 500 for Nana to CHF 900 for Mollie, suggesting that Nana is the most efficient producer (or has the lowest production costs) and Mollie the least efficient of the four.

The second part of the table relates these production costs to the market price and the quantity offered. When the price is below CHF 500, no seller is prepared to offer his houses, because the market price would not even cover the lowest production cost. This means that the producer's surplus would be negative, as sellers would incur losses at these prices.

As the price rises, sellers are prepared to offer increasing quantities of houses:

When the price is between CHF 500 and CHF 600, Nana is prepared to sell one house.
Between CHF 600 and CHF 800, Nana and Georgia together offer two houses.
Between CHF 800 and CHF 900, Nana, Georgia and Julie offer a total of three houses.
Finally, when the price exceeds CHF 900, all the sellers, including Mollie, are prepared to offer houses, with a total quantity offered of four houses.

The producer surplus for each seller is the difference between the market price and their cost of production. For example, if houses are sold for CHF 800, Georgia would have a surplus of CHF 200 (CHF 800 - CHF 600), Julie would have a surplus of CHF 0 (since her production cost is CHF 800), and Nana would have a surplus of CHF 300 (CHF 800 - CHF 500).

This information is crucial to understanding how price variations affect supply on the market. If the market price rises, this encourages more sellers to offer their product, as they can obtain a higher surplus. Conversely, a fall in prices could lead to a reduction in supply, as fewer sellers would find it profitable to sell their homes. This illustrates the law of supply, according to which the quantity offered of a good increases when its price rises, provided that all other factors remain constant.

Development of the Aggregate Supply Curve[modifier | modifier le wikicode]

The aggregate supply curve is an economic concept that represents the relationship between the price of a good or service and the total quantity of that good or service that all producers in the market are willing to sell. This curve is the result of adding together the different quantities that each individual producer is prepared to offer at each possible price level.

The aggregate supply curve is generally upward sloping, which means that the higher the price, the greater the quantities of the good or service that producers are willing to offer. This reflects the principle that higher prices can cover higher production costs and are therefore an incentive for producers to increase their output. At lower prices, fewer producers are able or willing to sell, as prices may not cover production costs or offer an acceptable profit margin.

The slope of the supply curve can vary according to a number of factors, such as production costs, technology, the number of sellers on the market, and producers' expectations for the future. Changes in these factors can shift the aggregate supply curve to the left or right. For example, an improvement in technology could reduce production costs and shift the supply curve to the right, indicating that a greater quantity is available at each price. Conversely, an increase in input costs could shift the curve to the left.

In a market, the aggregate supply curve interacts with the aggregate demand curve to determine the equilibrium price and the equilibrium quantity - the points at which the quantity producers are willing to sell equals the quantity consumers are willing to buy.

Understanding the aggregate supply curve is essential for market analysis, economic policy formulation and production decision-making. It is a fundamental representation of supply forces in the market and is used to predict producers' reactions to changes in market conditions.

The chart in question illustrates an aggregate supply curve for house painting services, highlighting the production costs of four separate suppliers. This supply curve is characterised by a series of staircases that indicate that each supplier enters the market at different price thresholds, corresponding to their individual production costs.

At the first level, we see that Nana is prepared to offer its painting services from a price of CHF 500, which corresponds to its cost of production. This suggests that Nana will only provide her services if the market price meets or exceeds this threshold, thereby covering her costs and potentially making a profit. As the market price rises to CHF 600, Georgia begins to offer her services, indicating that this is the point at which she can cover her costs and enter the market.

When the price reaches CHF 800, we see that Julie is also willing to provide her services, joining Nana and Georgia. This shows that Julie will only offer her services when the price is high enough to be profitable for her, given her production costs. Finally, Mollie's production cost of CHF 900 marks the highest threshold among suppliers, indicating that she will only enter the market when prices are high enough to exceed her higher production costs.

The aggregate supply curve, which rises step by step, underlines the economic principle that producers are prepared to sell more when the price rises, reflecting the law of supply. This curve visually represents the total quantity of painting services that suppliers are willing to offer at different price levels, and how supply increases as prices rise.

However, this representation does not take into account the complexity and dynamics of real competition in the market. Factors such as technological innovations, changes in raw material costs, or the entry of new competitors could influence the supply curve. For example, if a new technology reduced production costs for all suppliers, we could see the supply curve shift to the right, indicating a greater quantity offered at each price.

This chart helps to understand pricing decisions and production planning based on production costs and the price the market can bear. Suppliers need to carefully assess at what price they can profitably offer their services and how they can adjust their production in response to price changes to maximise their producer surplus.

Calculating Producer Surplus[modifier | modifier le wikicode]

Producer surplus is an economic concept which represents the difference between the price at which producers actually sell their goods or services on the market and the minimum price they would be prepared to accept for these goods or services, i.e. their cost of production. It is a measure of economic profit and indicates the level of welfare that producers derive from selling their products.

When a producer sells a product at a price that is higher than its production cost, he makes a surplus. This surplus can be considered as a gain or profit above what is necessary to induce the producer to produce the good. In other words, if the cost of production represents the minimum compensation required for the producer to be willing to supply a certain quantity of the good, any price above this cost of production contributes to the producer's surplus.

The total producer surplus in a market is the sum of the individual surpluses of all producers. It is graphically represented by the area above the supply curve and below the market price up to the quantity produced.

Producer surplus is an important analytical tool for economists and decision-makers. It can be affected by various factors, such as changes in production costs, technological innovations, government policies, or variations in market demand. For example, a tax on production may reduce the producer's surplus by increasing the cost of production, while subsidies may increase it.

In a market economy, the objective is often to maximise the combined surplus of consumers and producers, which is seen as an indication of economic efficiency. When markets operate freely without external intervention, and conditions of perfect competition are met, consumer and producer surplus is maximised, leading to an allocation of resources that is considered Pareto optimal.

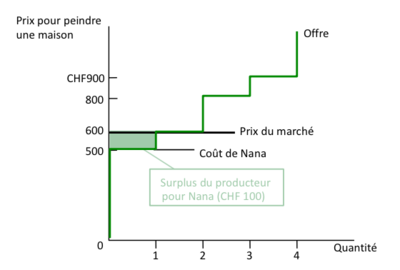
The graph represents a staircase supply curve for a house painting service and illustrates the producer surplus for one supplier, Nana, at a given market price. The staircase supply curve rises at each step, corresponding to the individual production costs of different suppliers to paint a house. These tiers indicate the price points at which additional suppliers enter the market. The higher the price, the more suppliers are willing to offer the service, as the price exceeds their respective production costs.

Nana's production cost is marked on the curve at CHF 500, which means that this is the minimum amount she should receive to cover her costs. The market price is currently set at CHF 600, which is represented by the horizontal line. The difference between the market price and Nana's production cost represents her producer surplus, which is visually indicated by the rectangular area below the market price and above Nana's production cost. In this case, Nana receives a producer surplus of CHF 100 for each house painted (CHF 600 - CHF 500). This surplus is the economic benefit she derives from selling her services above her costs. The graph shows that if the market price were below CHF 500, Nana would not be willing to provide the service because she would not be able to cover her production costs. Conversely, if the market price rose to, say, CHF 800, Nana's producer surplus would increase accordingly.

Producer surplus is a key element in understanding the motivation of producers and their response to price changes in the market. Suppliers will seek to maximise their producer surplus, which contributes to their overall profit. Changes in producer surplus can also indicate changes in the general well-being of producers, influencing their future investment and production decisions.

Producer surplus is represented graphically by the area between the supply curve and the market price, up to the quantity produced. Producer surplus is thus the total profit that producers make from selling their goods or services over and above their production costs. The supply curve itself indicates the minimum cost at which producers are willing to offer a certain quantity of the good or service. When the market price is higher than this minimum cost, producers benefit from a surplus, as they receive more than the minimum amount needed to cover their costs. The amount received by producers is the market price multiplied by the number of units sold, while the cost of production is generally represented by the supply curve. When you subtract the total cost of production (the area under the supply curve up to the quantity produced) from the total revenue (the product of the market price and the quantity sold), you get the producer surplus.

This concept is essential for understanding the distribution of profits in the economy and for assessing the efficiency of markets. In a situation of market equilibrium, the producer surplus, combined with the consumer surplus, can be used to assess the Pareto efficiency of the market, where no improvement in the welfare of one economic agent can be made without worsening the welfare of another.

Consequences of Changes on Producer Surplus[modifier | modifier le wikicode]

The change in producer surplus following a price change is given by the area above the supply curve between the two prices.

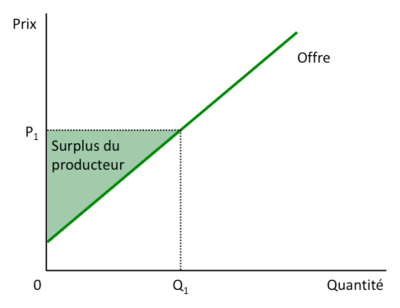
This graph illustrates a linear supply curve for a good or service on a specific market. This supply curve indicates that producers are prepared to offer a greater quantity of their good or service as the price increases, which is consistent with the law of supply in economics. The straight line rising from the origin shows this positive relationship between price and quantity offered.

The market price is represented by the horizontal line at P1, and the corresponding quantity that producers are willing to sell at this price is Q1. The producer surplus is then represented by the green-coloured area below price P1 and above the supply curve up to quantity Q1. This area illustrates the difference between the price producers receive for their goods or services and the cost of producing those goods or services. In other words, this green zone represents the net profit or gain that producers make over and above the minimum compensation they require to produce quantity Q1.

This producer surplus is a crucial element in understanding producers' economic incentives. The greater the surplus, the greater the incentive for producers to increase their production, as this means that they receive a price significantly higher than their cost of production. It is this profit that can be reinvested in the business for innovation, expansion, or used to increase the company's reserves. However, it is important to note that the graph represents a simplified situation. In reality, production costs may vary from one producer to another, and the supply curve may not be linear. In addition, changes in technology, input costs or government policies can shift the supply curve, affecting the producer surplus.

This graph serves as a model for analysing the impact of price variations on producers and can help in making strategic production and pricing decisions. It is also useful for policy makers who may consider interventions to stabilise prices or support certain industries, directly affecting producer surplus in the market.

Optimising Resource Allocation[modifier | modifier le wikicode]

Maximising Total Surplus[modifier | modifier le wikicode]

In a perfectly competitive market with no market failures, the market's allocation of resources can maximise overall welfare, known as total surplus, which is the sum of consumer surplus and producer surplus. Total surplus is a measure of economic efficiency and is maximised when markets operate freely and goods are traded until the additional surplus from each additional transaction is zero. Consumer surplus is the measure of the profit that consumers obtain by buying goods and services at a lower price than they would be willing to pay, while producer surplus is the profit that producers obtain by selling goods and services at a price higher than their cost of production. When these two surpluses are added together, they reflect the total market surplus.

The market is considered to be Pareto efficient when no other allocation of resources can make one individual better off without making another individual worse off. In such a market, the equilibrium price is reached when the quantity demanded equals the quantity offered, which also means that the total surplus is maximised. At this equilibrium point, it is not possible to increase the surplus of one side without decreasing that of the other. However, real markets can have failures that prevent such efficiency. These include the existence of market power (as in monopolies or oligopolies), externalities (where costs or benefits are not fully accounted for by the market), public goods (which are not efficiently produced or allocated by the market because of their non-excludable and non-rival nature) and imperfect information.

Where there are market failures, interventions such as regulations or tax policies may be necessary to correct these inefficiencies and move towards an allocation that maximises total surplus. Welfare economics is the study of these issues, seeking to understand how interventions can influence consumer and producer surplus and, consequently, overall welfare.

In an equilibrium market, the price that consumers pay for a good or service is equal to the price that producers receive for that good or service. Consequently, the price paid and the price received cancel each other out when the total surplus is calculated. This leads to a simplified formula for total surplus, which is the total value that consumers place on goods or services minus the total cost of producing those goods or services. This measure of total surplus is an indicator of the economic efficiency of the market.

When total surplus is maximised, there are no more possible transactions that could increase consumer value without proportionately increasing production costs, and vice versa. In such a state, the market is considered to be Pareto efficient, because it is not possible to make someone better off without making someone else worse off. In an ideal situation, the free market without intervention or failure will achieve this level of efficiency on its own. However, in reality, many markets experience failures that may require intervention to help maximise total surplus and improve economic efficiency. This may include corrections for externalities, regulations to counter market power, or the provision of public goods that the market alone would not optimally produce.

An allocation of resources is considered Pareto efficient if it maximises total surplus, which means that it is impossible to redistribute resources to make one person better off without making another worse off. In terms of surplus, this means that both the consumer surplus and the producer surplus are maximised and no additional gain can be made without one of the parties incurring a loss. In such an efficient situation, the market achieves what is known as a Pareto equilibrium, where resources are allocated in the most beneficial way for society as a whole. The total surplus, which is the sum of the consumer surplus and the producer surplus, is then at its highest level. This implies that consumers derive the greatest possible value from the goods and services they consume, and producers receive the best possible return on their investment and labour. Theoretically, this ideal is achieved in perfectly competitive markets where there are no externalities, public goods, information asymmetries or other market failures. In reality, public interventions such as regulations and taxes are often necessary to correct inefficiencies and move closer to Pareto efficiency.

This graph illustrates a typical market in equilibrium where the supply and demand curves cross. The supply curve is represented by an ascending line indicating that, as the price rises, producers are prepared to offer more goods because of the increased profitability. The demand curve, on the other hand, descends, showing that consumers are prepared to buy fewer goods as the price rises, in accordance with the law of demand.

The point where these two curves cross determines the equilibrium price of the market and the equilibrium quantity. At the equilibrium price, the quantity that producers are prepared to sell is exactly equal to the quantity that consumers are prepared to buy.

Consumer surplus is represented by the area coloured red below the equilibrium price and above the demand curve. This represents the difference between what consumers are prepared to pay (their perceived value of the good or service) and what they pay at the equilibrium price. It is the net profit that consumers obtain from their purchases on the market.

Producer surplus is the green area above the equilibrium price and below the supply curve. This surplus measures the difference between the market price and the price at which producers would theoretically be prepared to sell (which can be regarded as the cost of production). It is the net profit that producers make after selling their goods at the equilibrium price.

When the two surpluses are combined, they form the total market surplus, which is the measure of a market's economic efficiency. In a perfectly competitive market with no externalities or other market failures, total surplus is maximised at equilibrium. This means that it is not possible to increase the well-being of one individual without decreasing that of another, and that the market allocates resources as efficiently as possible.

The graph highlights the efficiency of the market in terms of optimising resources and maximising welfare. However, it should be noted that this ideal situation is based on a number of assumptions, including the absence of barriers to entry and exit, perfect information and homogeneous goods. In reality, these conditions are not always met, and inefficiencies may arise, sometimes requiring intervention to correct the market.

Case Study: Analysis of the Lamb Meat Market[modifier | modifier le wikicode]

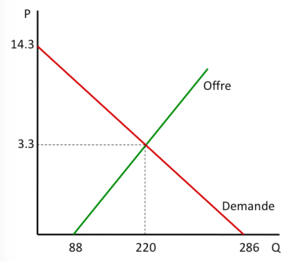
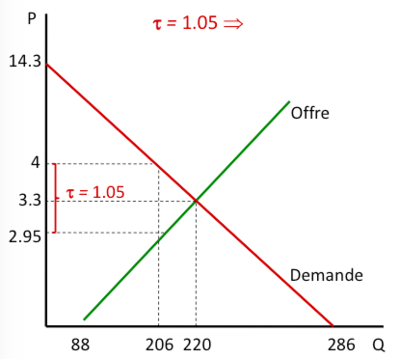
The following graph represents a lamb meat market with the supply and demand curves intersecting to indicate market equilibrium. The vertical axis (P) represents the price of lamb in dollars and the horizontal axis (Q) represents the quantity of lamb.

The demand curve, represented by the red line descending from left to right, indicates that the lower the price, the greater the quantity demanded. This reflects the law of demand: consumers generally demand more of a good as its price falls. The demand curve crosses the price axis at 14.3, which would be the maximum price consumers would be prepared to pay for zero quantity of the product, and it crosses the quantity axis at 286, which represents the maximum quantity consumers would take if lamb meat were free.

The supply curve, represented by the green line rising from left to right, shows that the higher the price, the greater the quantity offered. This follows the law of supply: producers are prepared to sell more of a good as its price rises. The supply curve intersects the quantity axis at 88, which would be the minimum quantity offered at a price of 0.

The two curves intersect at the equilibrium point, where the price is 3.3 dollars and the equilibrium quantity is 220 units. This point of intersection indicates the price and quantity where the quantity that consumers want to buy is exactly equal to the quantity that producers want to sell.

This graph illustrates a market in equilibrium with no surplus or shortage of lamb. The equilibrium is Pareto efficient, which means that it is not possible to make someone better off without making someone else worse off. If the price were higher than 3.3 dollars, there would be a surplus of lamb because the quantity offered would exceed the quantity demanded. If the price were below 3.3 dollars, there would be a shortage because the quantity demanded would exceed the quantity offered. In such a market, the total surplus (the sum of the consumer and producer surpluses) is maximised at this equilibrium point. Consumer surplus would be the area below the demand curve and above the equilibrium price, while producer surplus would be the area above the supply curve and below the equilibrium price. Their sum represents the total economic benefits generated by the market for all participants.

q_{a}^{d}=286-20p_{a}^{d}

q_{a}^{s}=88+40p_{a}^{s}

q^{*}=220

et

p^{*}=3.3\$

SC={\frac {(14.3-3.3)\times 220}{2}}={\frac {2420}{2}}=1210

SP={\frac {220+88}{2}}\times 3.3=508.2

Stot=SC+SP=1210+508.2=1718.2

In this example, the lamb meat market is analysed using linear functions to represent demand and supply. The demand and supply equations are given by $q_{a}^{d}=286-20p_{a}^{d}$ and $q_{a}^{s}=88+40p_{a}^{s}$ respectively, where $p_{a}^{d}$ and $p_{a}^{s}$ are the demand and supply prices. In equilibrium, the quantity demanded and the quantity offered are equal to $q^{*}=220$ , and the equilibrium price is $p^{*}=3.3$ dollars.

According to the equations provided:

The equilibrium quantity ( $q^{*}$ ) is 220 units.

The equilibrium price ( $p^{*}$ ) is 3.3 dollars.

Consumer surplus (CS) is calculated as the area of a triangle below the demand curve and above the equilibrium price. Mathematically, it is calculated as the difference between the maximum willingness to pay (the price intercept on the price axis of the demand function when quantity is zero) and the equilibrium price, multiplied by the quantity sold in equilibrium, all divided by two. In this example, the consumer surplus is $SC={\frac {(14.3-3.3)\times 220}{2}}={\frac {2420}{2}}=1210$ dollars.

Producer surplus (PS) is also calculated as the area of a triangle, but this time above the supply curve and below the equilibrium price. It represents the difference between the equilibrium price and the price at which producers would be willing to supply the good for zero units (the cost of production for zero units), multiplied by the quantity sold at equilibrium, all divided by two. The producer surplus is $SP={\frac {(220+88)}{2}}\times 3.3=508.2$ dollars.

Finally, total market surplus (Stot) is the sum of consumer surplus and producer surplus. It represents the total sum of economic benefits for consumers and producers in the lamb meat market. Here, the total surplus is $Stot=SC+SP=1210+508.2=1718.2$ dollars. This means that the allocation of resources in this lamb meat market, at the equilibrium price and quantity, generates a total economic welfare of 1718.2 dollars for society.

Market Efficiency Discussion[modifier | modifier le wikicode]

Key Concepts of Market Efficiency[modifier | modifier le wikicode]

The fundamental principles of economic theory concerning the efficiency of markets, particularly in a framework of perfect competition. Here is a detailed explanation of each of these remarks:

Allocation to buyers according to value assigned: In an efficient market, goods and services are allocated in priority to the buyers who value them most. This is demonstrated by their willingness to pay a higher price than other buyers. This principle ensures that resources are used in the most advantageous way, as goods are consumed by those who derive the greatest subjective benefit from them. This allocation can be seen on the market equilibrium graph, where the price reflects the meeting between consumers' willingness to pay and producers' willingness to sell.
Allocation to the most efficient producers : Producers who can offer goods and services at the lowest cost, thanks to advantages such as more efficient technologies, better access to resources, or more efficient production methods, will be the ones who can sell at competitive prices on the market. This leads to a situation where the most efficient producers are those who stay in business, while the least efficient leave the market or adapt to become more competitive. This maximises productive efficiency, as goods are produced at the lowest possible cost.
Total surplus maximisation and laissez-faire optimality: The idea that the free market, without external intervention, leads to an allocation of resources that maximises total surplus is a conclusion of economic welfare theory. In the absence of market failures, the competitive equilibrium achieved is Pareto efficient, because it is not possible to improve the well-being of one individual without worsening that of another. This laissez-faire principle is often cited in defence of free markets and economic policies that limit government intervention.

These remarks presuppose a set of idealised assumptions that include perfectly competitive markets, an absence of market power for buyers and sellers, the absence of externalities, complete and perfectly symmetrical information, and well-defined and enforced property rights. In reality, these conditions are often not all met, which may justify intervention to correct the resulting inefficiencies and improve overall well-being.

Efficiency in the Pareto sense, which is achieved in a perfectly competitive market, focuses solely on maximising the total surplus without taking into account the distribution of this surplus between the various market players. In other words, although a market may be efficient by maximising total surplus, this does not guarantee that the result is equitable or 'fair' in terms of the distribution of resources and income between individuals.

Equity is a normative concept that concerns social justice and the distribution of goods and wealth in society. Criteria for fairness vary widely according to political and philosophical perspectives, and what is considered fair in one society may be perceived differently in another. For example, a distribution of resources that promotes equality of outcomes may be considered fair according to some ethical frameworks, while others might value equality of opportunity or proportionality, where rewards are distributed according to each individual's contribution.

Economic efficiency and equity are therefore often treated separately in economics. Public intervention, such as progressive taxation and income redistribution, is commonly used to correct inequality and improve equity. However, these interventions can sometimes conflict with market efficiency by introducing distortions. As a result, policymakers face the challenge of balancing efficiency and equity, which may require delicate trade-offs and policy choices.

Exploring the Limits of Market Autonomy[modifier | modifier le wikicode]

The limits of the laissez-faire principle arise from a variety of real-world conditions that deviate from the ideal of perfectly competitive markets. These market failures often justify government intervention to correct or mitigate inefficiencies and promote a more equitable allocation of resources. The main market failures mentioned are

Imperfect competition: In a market where there is imperfect competition, such as monopolies, oligopolies or monopsomes, producers or consumers can influence prices and quantities, preventing the market from achieving an efficient allocation of resources. Market power can lead to higher prices and lower quantities than would be achieved in a competitive market, reducing the total surplus.
Positive or negative externalities: An externality is an effect that an economic transaction between two parties has on a third party who is not directly involved in the transaction. Negative externalities, such as pollution, generate social costs that are not taken into account by producers or consumers, while positive externalities, such as innovation, generate social benefits that exceed private benefits. Markets left to their own devices fail to internalise these costs or benefits, which can lead to overproduction in the case of negative externalities and underproduction in the case of positive externalities.
Public goods: Public goods are goods that are non-excludable (no one can be prevented from consuming them) and non-rivalrous (consumption by one person does not affect availability to others). Examples include national defence, lighthouses or radio broadcasts. Markets tend to under-produce public goods because it is difficult to force users to pay for their consumption, leading to a free rider problem
Inequality problems: Laissez-faire does not guarantee a fair distribution of wealth or income. Markets can lead to outcomes where wealth is concentrated in the hands of a few, while others may not have sufficient resources to meet their basic needs. This inequality may be due to initial differences in resource endowments, education or capabilities.

In each of these cases, it may be necessary for governments to intervene with policies such as regulation, taxation, provision of public goods and redistribution programmes to correct market failures and promote a fairer and more efficient society.

Analysis of Government Interventions and Failures[modifier | modifier le wikicode]

Just as there are market failures, there are also government failures, sometimes referred to as 'state failures'. These failures can occur when government interventions fail to improve market outcomes or unintentionally worsen them. Examples of such failures include

Bureaucratic inefficiency: Governments can suffer from red tape that hampers their ability to respond quickly and effectively to economic problems. Bureaucracy can be inefficient because of the complexity of procedures, the lack of performance incentives, or the difficulty of measuring and rewarding productivity.
Imperfect information: Like market participants, government decision-makers may lack complete or accurate information, which can lead to sub-optimal decisions or unintended consequences. Regulatory capture: This occurs when regulated industries exert undue influence on the government agencies responsible for regulating them, often to shape laws and policies to their advantage. This can result in regulations that serve the interests of business at the expense of the public interest.
Short-term political objectives: Politicians may be motivated by short-term electoral cycles, leading them to focus on policies that generate visible short-term benefits at the expense of long-term benefits, or to avoid unpopular but necessary measures.
Incentive problems: Incentives for government actors are not always aligned with the interests of society as a whole. For example, politicians may have an incentive to engage in excessive public spending to win popular support, even if this spending is not economically justified.
High transaction costs: Government intervention is often accompanied by high transaction costs, particularly in terms of implementation and regulatory compliance.

For these and other reasons, it is crucial to carefully evaluate any planned government intervention to ensure that it achieves its objectives without causing collateral damage or unexpected side-effects. Cost-benefit analysis is an important tool in this process, weighing the expected benefits of a policy against its potential costs and risks of failure.

Theory and Practice of Taxation[modifier | modifier le wikicode]

Introduction to the Principles of Taxation[modifier | modifier le wikicode]

When a government imposes a tax on a good or service, it has an immediate and significant impact on the economy of that market. The presence of the tax tends to reduce the quantity of goods or services traded, as it introduces an additional burden that must be taken into account by consumers and producers. For example, if a tax is imposed on fuel, consumers may reduce their consumption because of the increase in price, and service stations may be less inclined to keep large quantities of fuel in stock, knowing that demand has fallen.

The price that consumers pay for the taxed good or service generally increases by the value of the tax. Let's take the example of fuel again: if the government imposes a tax of $0.20 per litre, the price consumers pay at the pump will probably increase by the same amount, making fuel more expensive for drivers. On the other hand, the price producers receive for each unit sold falls, because some of the money that would otherwise have gone to them now goes to the government in the form of tax. In our example, this could mean that oil refineries receive less for each litre of fuel sold, which could lead them to reduce production.

A key aspect of taxation is that the tax incidence, or the actual economic burden of the tax, does not necessarily depend on who is legally required to pay it. Whether the tax is levied on sellers or buyers, the effects on the market in terms of prices and quantities will be the same after market adjustments have taken place. This is due to changes in the behaviour of consumers and producers in response to the tax, which ultimately affect equilibrium prices and the distribution of the tax burden between them.

Who bears the actual burden of the tax depends largely on the elasticities of supply and demand. Elasticity measures the sensitivity of the quantity demanded or offered to a change in price. If, for example, we consider an essential drug for which there is no close substitute and which patients absolutely need, demand is likely to be inelastic. In this case, even if the price of the drug rises as a result of the tax, patients will continue to buy it because they have no other choice. As a result, consumers will end up bearing a large proportion of the tax. Conversely, if a product has many close substitutes, such as fruit, a small price increase due to a tax could lead consumers to switch to the alternatives, meaning that producers cannot pass on the full tax to consumers without losing a significant share of their market.

Taxation has a two-fold impact on the economy: it affects the welfare of market participants and generates revenue for the government.

Impact on welfare (Total Surplus)

The introduction of a tax into a market reduces the total surplus, which is the sum of the consumer surplus (CS) and the producer surplus (PS). The mechanism is as follows:

Consumer Surplus: The tax increases the price consumers pay for the good or service, which reduces their surplus. They pay more for each unit, and some consumers may choose not to buy the good because of its extra cost, losing the utility they would otherwise have derived from consuming it.
Producer surplus: At the same time, the tax reduces the net price producers receive after tax. This reduces their incentives to produce and sell, which in turn reduces their surplus. The reduction in the quantities produced and sold can also lead to a loss of productive efficiency.

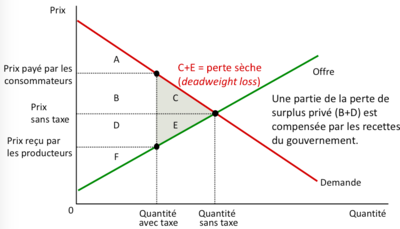
The combined loss of consumer and producer surplus is often illustrated by a triangle of deadweight loss on a supply and demand graph. This deadweight loss represents the loss of economic welfare that does not benefit consumers, producers or even the government: it is simply a loss of efficiency due to the tax.

Government revenue (GS)

On the other hand, the tax generates revenue for the government (SG), which is the product of the tax per unit multiplied by the number of units sold after the tax is imposed. This money can be used to fund public services, investment in infrastructure, education and health, or to redistribute resources as part of social programmes. So, although taxation reduces the total market surplus, it allows government functions to be carried out which can, in turn, have a positive impact on social well-being.

The overall effectiveness of a tax, in terms of the trade-off between the loss of market welfare and the benefits of government spending, depends on how the funds are used. If tax revenues are spent in a way that improves overall welfare or corrects other market inefficiencies (such as negative externalities), the net impact of taxation can be positive. On the other hand, if revenues are mismanaged or spent inefficiently, the net effect may be a decrease in social welfare.

In real life, the design of tax systems seeks to balance these two aspects: maximising government revenues while minimising distortions and welfare losses in markets. Governments must therefore carefully consider tax rates and application methods, taking into account the elasticities of supply and demand to minimise the negative impact on production and consumption. Public finance focuses on identifying the most appropriate tax structure to generate the necessary government revenues while minimising the negative impact on the economy. A key principle is to avoid major disruptions in consumer and producer behaviour, as significant changes can lead to a loss of economic efficiency, known as deadweight loss. This loss represents the value of trade that does not occur because of the tax and that would otherwise have benefited both consumers and producers.

To minimise this social cost, economists often suggest imposing taxes on goods and services whose demand or supply is inelastic, i.e. those for which price variations do not substantially change the quantity demanded or offered. For example, taxes on tobacco products are generally effective because even with a significant increase in prices due to taxation, demand falls little. Similarly, taxes on petrol tend to generate stable revenues because motorists make few changes to their driving behaviour in response to short-term price variations.

The optimal approach to taxation also seeks to reflect marginal social costs. So if the production or consumption of a good causes negative externalities, such as pollution, the tax should be adjusted to include these costs, bringing the market price into line with the true cost to society. This can not only deter harmful activities but also generate revenue that can be used to mitigate the damage caused. Fairness is also an important consideration. A fair tax system is often measured by its progressivity, i.e. its ability to impose heavier tax burdens on those with a greater ability to pay. Progressive taxes, which increase as a percentage with income level, aim to spread the tax burden more evenly across society. This can be seen in income tax systems where higher marginal rates are applied to higher income brackets.

Finally, administrative efficiency is essential for a good tax structure. Tax systems must be designed to be simple to understand and easy to administer. Complex systems can not only generate high administrative costs but also encourage tax evasion, thereby reducing the efficiency of tax collection. Efforts to combat tax evasion and improve tax compliance are examples of how governments try to maximise revenues while minimising costs. Taken together, these principles guide the creation of tax policies aimed at balancing revenue needs with the objectives of maintaining a healthy and equitable economy. The success of these policies is often measured by their ability to support public spending without imposing excessive tax burdens that could inhibit economic growth or exacerbate inequality.

Analysis of the Social Cost of Taxes[modifier | modifier le wikicode]

An important principle about taxation and its impact on the economy is that when a tax is imposed on a good or service, regardless of whether it is legally imposed on consumers or producers, it has the effect of reducing the size of the market and affects prices and quantities in the same way.

In a free market, prices and quantities are determined by the interaction of demand and supply. When a tax is introduced, it creates a gap between the price consumers pay and the price producers receive. This gap is the tax itself. If the tax is imposed on consumers, the price they pay increases. If it is imposed on producers, the price they receive for each unit sold falls. In both cases, the net effect is a reduction in the quantity traded on the market.

The mechanism behind this can be explained as follows:

When consumers are taxed, they have to pay more for each unit of the good or service, which leads them to demand less;
When producers are taxed, they receive less money for each unit sold, which leads them to offer less on the market.

In both cases, the total quantity of goods traded falls. This result is due to the fact that the tax increases the total cost of purchase for consumers and reduces the net income for producers, leading to a reduction in the quantity demanded and offered. This is known as the tax burden, which is shared between consumers and producers, regardless of which party formally pays the tax.

In practice, the exact impact of the tax on the prices paid by consumers and received by producers depends on the relative elasticities of demand and supply. If demand is relatively inelastic (consumers do not reduce their consumption much in response to a price increase), they will bear a greater share of the burden of the tax. Conversely, if supply is relatively inelastic (producers do not reduce their supply much in response to a fall in prices), producers will bear a greater share of the burden.

This concept is fundamental in economics for understanding how taxes influence markets and for assessing the impact of tax policies. It also highlights the fact that tax decisions cannot be taken lightly, as even seemingly targeted taxes can have widespread effects throughout the economy.

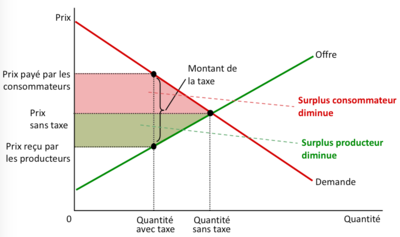
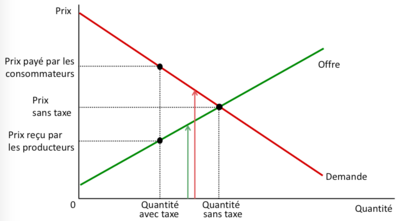
This graph presents a typical market with the introduction of a tax and shows the impact on consumer and producer surplus as well as on prices and quantities. The supply (green) and demand (red) curves intersect at the equilibrium point without tax, indicating the quantity and price where the quantity demanded by consumers corresponds exactly to the quantity that producers wish to sell. Consumer surplus is represented by the area below the demand curve and above the equilibrium price, and producer surplus is the area above the supply curve and below the equilibrium price.

The introduction of the tax creates a gap between the price paid by consumers and the price received by producers, represented by the vertical space between the two prices on the graph. This area corresponds to the amount of tax. After taxation, the new equilibrium point shows that the quantity traded decreases (the "Quantity with tax" is less than the "Quantity without tax"). The price paid by consumers increases, while the price received by producers decreases. The difference between these two prices is the amount of tax levied by the State.

The consumer surplus is reduced because consumers pay a higher price and buy fewer goods. Similarly, the producer's surplus is reduced because they receive a lower price for the goods they sell and sell a smaller quantity. The coloured areas represent the reduction in surplus for consumers (red) and producers (green).

The deadweight loss, or efficiency cost, is illustrated but not explicitly marked on this graph. It corresponds to the area of the triangle formed between the supply and demand curves and the new quantity and price lines after taxation. This deadweight loss represents the lost economic welfare that is not compensated for by consumers or producers, or even by government revenue from the tax.

This graph clearly illustrates that the tax leads to a loss of efficiency in the market by causing a reduction in the beneficial exchanges that would have taken place in the absence of the tax. However, it is essential to note that the government revenues generated by this tax can be used to finance public services or policies that improve social welfare. The overall effectiveness of this tax would therefore depend on how the revenues are used and the impact they have on society as a whole.

Exploring Tax Revenues[modifier | modifier le wikicode]

The graph shows how a tax introduced into a market affects not only consumer and producer surplus, but also how it generates revenue for the government. This government revenue is represented by the rectangle between the two new price lines after taxation (the price paid by consumers and the price received by producers).

To calculate government revenue (GR), we multiply the amount of tax per unit (the vertical difference between the price paid by consumers and the price received by producers) by the quantity of goods traded on the market after the introduction of the tax. This is the area of the rectangle mentioned above.

The amount collected in this way can be substantial, depending on the size of the tax and the quantity of goods traded. However, governments need to be careful when setting the tax rate, because taxes that are too high can considerably reduce trade in the market and thus reduce tax revenues, a phenomenon known as the Laffer effect. So there is a balance to be struck between maximising revenues without causing an excessive contraction in economic activity.

In real life, the revenue collected through taxes can be allocated to various public purposes, such as education, health, defence, or to fund transfer programmes aimed at reducing inequality. How this revenue is spent can in turn influence a nation's economic and social well-being. Prudent and effective fiscal and budgetary management is therefore crucial to ensure that the benefits of raising taxes outweigh the efficiency costs they impose.

This graph shows the impact of a tax on a market. We see two different prices: the higher price that consumers pay after the introduction of the tax, and the lower price that producers receive for their goods or services. The difference between these two prices is the amount of tax per unit.

The vertical line that crosses the supply and demand curves marks the quantity traded after the imposition of the tax, while the vertical dotted line further to the right indicates the quantity that would have been traded in the absence of the tax. The quantity traded decreases as a result of the tax, which is a direct manifestation of the decrease in market efficiency: fewer transactions take place because of the additional cost imposed by the tax.

The beige rectangle between the two prices indicates the government revenue generated by the tax. This revenue is calculated by multiplying the amount of the tax by the quantity of goods traded after the introduction of the tax. This is a source of revenue for the government, but it comes at the price of a loss of efficiency for the market.

It is important to note that both consumer surplus and producer surplus are reduced by the tax. Consumer surplus is reduced because consumers pay a higher price, and producer surplus is reduced because producers receive a lower price. This reduction in surplus is represented by the decrease in the areas below the demand curve for consumers and above the supply curve for producers.

This graph clearly illustrates the concept of deadweight loss, which is the loss of economic welfare that is not recovered by tax revenues. The dry loss would be located between the supply and demand curves and the new quantity traded, illustrating the transactions that no longer occur because of the tax. In short, although the tax generates revenue for the government, it also reduces market efficiency by reducing the quantity of goods traded and reducing consumer and producer surpluses. Governments must therefore carefully weigh the benefits of tax revenues against the efficiency costs they impose on the market.

Evaluating the Net Effect of Taxation on Surplus[modifier | modifier le wikicode]

The net effect of a tax on total surplus, which is the sum of consumer surplus and producer surplus, is generally negative. When the tax is introduced, as illustrated in the graph, it creates a distortion in the market that leads to a loss of economic efficiency.

Here's what happens:

Higher price for consumers: Consumers face a higher price because of the tax. This reduces their surplus, because they have to pay more for each unit of the good or service, and some consumers who would have bought at the equilibrium price without tax choose not to buy at the higher price. Consumer surplus is therefore reduced by the area between the equilibrium price without tax and the higher price with tax, up to the new quantity traded after taxation.
Lower price for producers: Producers receive a lower price for each unit sold, which is lower than the equilibrium price without tax because of the tax that must be paid to the government. This reduces their incentive to produce and offers less on the market, which reduces the producer surplus. The producer surplus is therefore reduced by the area between the equilibrium price without tax and the price after tax received by producers, up to the new quantity traded.
Deadweight loss: The reduction in quantities traded leads to a deadweight loss. This is the loss of economic well-being that benefits neither consumers nor producers, nor does it constitute income for the government. It represents the transactions that would have taken place in the absence of the tax and that would have increased the well-being of consumers and producers. This loss is represented by the triangle formed by the difference between the quantities traded with and without tax and between the supply and demand curves.
Government income : As the consumer and producer surplus falls, the government generates revenue from the tax, equivalent to the tax per unit multiplied by the quantity traded after the tax. This revenue can be used for public services that can, in theory, increase the well-being of society as a whole.

The net effect on total surplus is therefore the sum of the reduced consumer and producer surplus minus the deadweight loss, which can be partially offset by the value of the public services financed by the tax revenues. If the use of tax revenues is less efficient than the loss of surplus due to the tax, the net effect will be a reduction in economic welfare. If, on the other hand, the tax revenues are used in a way that significantly improves welfare (for example, by funding essential public goods or improving equity), the net effect may be positive. In most cases, however, taxation results in a net loss of surplus, as the gains from government revenues generally do not fully compensate for the loss of market efficiency.

The table provided summarises the impact of taxation on consumer surplus, producer surplus and tax revenue, as well as the net effect on total surplus, which is the sum of consumer and producer surplus plus tax revenue.

Without tax:

Consumer surplus is represented by the sum of areas A, B and C.
Producer surplus is represented by the sum of areas D, E and F.
Since there is no tax, there is no tax revenue.
Total surplus is the sum of consumer and producer surplus (A + B + C + D + E + F).

With Tax:

Consumer surplus is reduced to area A only, indicating a loss of surplus equivalent to areas B and C.
Producer surplus is now represented by area F only, reflecting a loss of surplus equivalent to areas D and E.
Tax revenue generated by tax is represented by areas B and D.
Total surplus with tax is now the sum of A, B, D and F.

Change:

Consumer surplus decreases by (B + C)
Producer surplus decreases by (D + E)
Tax revenue is (B + D), which is the new government revenue due to the tax
Total surplus decreases by (C + E), representing the deadweight loss due to the tax.

The deadweight loss (C + E) represents the efficiency cost of the tax, i.e. the loss of economic welfare that is not offset by consumer surplus, producer surplus or tax revenue. This is a net loss for society because transactions that would have benefited both consumers and producers (and therefore the economy as a whole) are no longer taking place. The presence of this deadweight loss is the main reason why taxation can reduce the overall efficiency of the market, even if it is necessary to finance public spending.

Tax revenue (B + D) is what the government collects and can potentially use to finance public goods or services, redistribution programmes, and other government functions. The overall effectiveness of the tax therefore depends largely on how this revenue is used. If the government spends them in a way that creates value equal to or greater than the deadweight loss, then the net effect on social welfare can be neutral or even positive. However, this is difficult to achieve in practice and depends very much on the government's priorities and efficiency in allocating tax resources.

This graph shows a typical market where a tax has been introduced, resulting in a difference between the price paid by consumers and the price received by producers. The coloured and marked areas (A, B, C, D, E, F) represent different parts of the consumer and producer surplus and the impact of the tax. The coloured and marked areas (A, B, C, D, E, F) represent different parts of the consumer and producer surplus as well as the impact of the tax. Before the tax, the market is in equilibrium at the "Quantity without tax" where the demand curve (red) and the supply curve (green) cross. At this stage, consumer surplus is represented by areas A, B and C, and producer surplus by areas D, E and F.

After taxation, the new price that consumers pay and the new price that producers receive create a division in these surpluses:

Consumer surplus is now represented only by area A, showing a loss of surplus equivalent to areas B and C.
Producer surplus is reduced to area F, with a loss of surplus corresponding to areas D and E.
Area B plus area D equals the government revenue generated by the tax. This indicates that although the private surplus (consumers and producers) has decreased, the government now receives income that can be used to finance public services or other government objectives.
Areas C and E combined represent the deadweight loss or efficiency cost. This dry loss is the irreversible loss of economic welfare and reflects the transactions that no longer occur because of the tax. These transactions would have provided more value than their cost in resources and would therefore have increased overall economic well-being.

The dry loss (C+E) is of particular concern because it represents a net loss to society: it is not compensated by any party and does not contribute to tax revenues. This is why economists often prefer taxes that minimise the deadweight loss, i.e. taxes on goods with inelastic demand or supply. In conclusion, the graph illustrates that even if government revenue is increased by the tax, the cost to society in terms of lost economic surplus is unavoidable. The effectiveness of such a tax must therefore be assessed not only in terms of the revenue collected but also by considering the effect on society's overall well-being.

Intuitive Understanding of Tax Impacts[modifier | modifier le wikicode]

In a tax-free market, consumers and producers engage in mutually beneficial exchanges. Consumer surplus is the difference between what consumers are prepared to pay for a good or service (their profit) and the price they pay. The producer surplus is the difference between the selling price and the cost of production. These accumulated surpluses represent total welfare or total market surplus.

When a tax is introduced, it eliminates some of these beneficial exchanges. More precisely, the exchanges that would have taken place between the price without tax and the price with tax no longer occur. This means that the benefit that consumers would have derived from these exchanges (represented by the consumer surplus) and which is greater than the cost of production (represented by the producer surplus) is not realised. These unrealized transactions, which would have generated a surplus for both parties, are lost because of the tax, and this lost surplus is known as the deadweight loss.

By imposing the tax, the government collects revenue on the transactions that continue to take place after the tax, but not on the deadweight loss. The tax revenue is therefore lower than it would have been if these exchanges had taken place. The deadweight loss is therefore a net loss for society because it represents value that is not captured by anyone: neither consumers nor producers, nor is it recovered in the form of tax revenue by the government. This is why economists often recommend taxes that minimise the deadweight loss, particularly in cases where the goods or services are essential and reducing transactions can have particularly harmful consequences for social well-being.

Lhis graph illustrates the effects of a tax on a market, assuming one tax is levied per unit of good or service traded. The demand and supply curves represent consumers' willingness to pay and producers' cost of production respectively.

Before the tax is imposed, the market reaches a point of equilibrium where the quantity demanded by consumers coincides with the quantity offered by producers, at a certain price. This equilibrium is indicated by the intersection of the demand and supply curves.

After the tax, there is a difference between the price paid by consumers and the price received by producers, which is equivalent to the amount of tax per unit. This difference creates a new equilibrium point where the quantity traded is reduced (indicated by "Quantity with tax"), and the market no longer functions optimally. The effects of this taxation are as follows:

For consumers: They pay a higher price for the good or service, which reduces their surplus (the difference between what they are willing to pay and what they actually pay)
For producers: They receive a lower price for each unit sold, which also reduces their surplus (the difference between the selling price and the cost of production).

The red vertical line indicates the quantity traded without tax, and the green vertical line indicates the quantity traded with tax. The difference between these two quantities represents the deadweight loss due to the tax. This shows the reduction in the number of transactions that would be mutually beneficial and that no longer occur because of the tax.

The deadweight loss, i.e. the total loss of economic welfare, is not explicitly represented on this graph but would lie between the two quantities and below the demand curve and above the supply curve. It represents the surplus that consumers and producers would have received without the tax and which is not captured by government revenues.

Government revenue comes from the tax collected on transactions that continue to occur after taxation. Although the government earns revenue, the deadweight loss indicates that the cost to society in terms of unrealised transactions is a direct negative effect of the tax.

The net effect of taxation is therefore a reduction in total market surplus, consisting of a reduction in consumer and producer surplus, as well as a loss of economic efficiency, although the government receives new revenue. This suggests that the objective of any tax policy should be to strike a balance between the need for public funding and the minimisation of tax-induced economic distortions.

Case Study: Taxation in the Lamb Meat Market[modifier | modifier le wikicode]

The graph below represents a market for lamb meat, with a tax τ imposed on the product. The descending demand curve indicates that, as the price increases, the quantity demanded of lamb meat decreases, in accordance with the law of demand. The rising supply curve shows that, as the price increases, the quantity of lamb meat offered also increases, which is typical according to the law of supply.

The tax-free price is the point where the supply and demand curves intersect, indicating the equilibrium price and equilibrium quantity in a tax-free market. However, with the introduction of a tax per unit of τ=1.05, the effective price for consumers increases, while the price received by producers decreases.

The effects of introducing the tax are as follows:

Consumer Price: The price paid by consumers for lamb meat increases by more than 4 units of money. This results in a decrease in the quantity demanded of lamb, as indicated by the shift to the left of the equilibrium quantity from 220 to 206 units.
Producer price: Although the market price for consumers is higher than 4, the effective price received by producers is lower than 3.3, after deduction of the tax. This may reduce the incentive for producers to produce and offer lamb meat.
Government revenue: The tax generates revenue for the government, which is the product of the tax per unit (τ) multiplied by the quantity sold after the application of the tax (206 units in this case).

What is important to note here is the existence of an economic welfare loss, or deadweight loss, that results from the quantity of lamb meat that is no longer traded due to the tax. Before the tax, the market would trade 220 units, but with the tax, only 206 units are traded, which means that 14 units that would have been beneficial to consumers and producers are no longer traded. This loss of mutually beneficial exchange is the deadweight loss that is not offset by any government revenue.

In short, while the tax generates revenue for the government, it also reduces the consumer and producer surplus and leads to a loss of economic efficiency due to the reduction in market exchanges. This shows the challenge inherent in imposing taxes: striking a balance between the need to fund public spending and minimising the negative impact on the economy.

q_{a}^{d}=286-40(p_{a}^{s}+1.05)=88+40p_{a}^{s}=q_{a}^{s}

q_{a}^{s}=2.95\$

,

p_{a}^{d}=4\$

et

q^{*}=206

SG=1.05\times 206=216.3

SC={\frac {(14.3-4)\times 206}{2}}=1060.9

\Delta SC=1060.9-1210=-149.1

SP=433.65-508.2=-74.55

Stot=SG+SC+SP=1710.85

\Delta Stot=216.3-149.1-74.55=-7.35

{\text{dead load}}={\frac {(4-2.95)(220-206)}{2}}=7.35

The producer surplus after the tax is calculated as $SP={\frac {(2.95+1.05)\times 206}{2}}=\$412.00$ .

The change in producer surplus compared with the situation without tax is: $\Delta SP=412.00-508.2=-96.2\$$ .

The consumer surplus after the tax is calculated using the formula: $SC={\frac {(14.3-4)\times 206}{2}}=\$1060.9$ .

The change in consumer surplus is therefore: $\Delta SC=1060.9-1210=-\$149.1$ .

Government revenue is calculated by: $SG=1.05\times 206=216.3\$$ .

The total surplus with tax, which is the sum of consumer surplus, producer surplus after tax and government revenue, is: $Stot=SG+SC+SP=216.3+1060.9+412.00=\$1689.2$ .

The change in total surplus compared to the situation without tax is: $\Delta Stot=216.3-149.1-96.2=-29.0\$$ .

The deadweight loss, which is the loss of economic welfare due to the tax and represents trade that no longer occurs, is calculated as the area of the triangle formed by the difference in prices and quantities traded: ${\text{dead load}}={\frac {(4-2.95)\times (220-206)}{2}}=7.35\$$ .

These calculations show that the tax caused a reduction in consumer and producer surplus, and introduced a deadweight loss into the economy. Although the government collected revenues of $216.3, the total loss in surplus for consumers and producers was $149.1 and $96.2 respectively, leading to a net loss in total surplus of $29.0. The deadweight loss represents the irrecoverable loss of economic efficiency, and is exactly equal to the reduction in total surplus of $7.35, confirming that private surplus losses are not fully compensated by tax revenues.

Impact of Elasticity on Taxation[modifier | modifier le wikicode]

The impact of a tax on the economy depends very much on how consumers and producers react to price changes, which is measured by the price elasticities of demand and supply.

When demand or supply is elastic, this means that the quantity demanded or offered changes considerably in response to a change in price. If a tax is introduced in such a context, consumers react by significantly reducing their demand, or producers by reducing their supply, because of the increase in the price of the good or service being taxed. This leads to a sharp fall in the quantities traded on the market. The significant reduction in the quantities traded leads to an increase in the social cost of the tax, as more mutually beneficial trade is avoided. In other words, the dry loss (the area C+E in the previous graph) increases with the elasticity of demand or supply.

Conversely, when demand or supply is inelastic, consumers or producers make little change in the quantity demanded or offered in response to a change in price. Consequently, the introduction of a tax in a market characterised by low elasticity leads to a small reduction in the quantities traded and therefore a lower social cost. The deadweight loss is lower because fewer transactions are lost.

To maximise the efficiency of revenue collection, it is in the government's interest to impose taxes on goods and services for which demand and supply are relatively inelastic. By taxing these markets, the government can collect revenue without causing a significant reduction in the quantities traded, thereby minimising the deadweight loss and the social cost of taxation. This explains why many governments impose high taxes on products such as tobacco and petrol, where demand tends to be relatively inelastic: even if the price rises as a result of the tax, the reduction in consumption is relatively small, making it possible to collect significant tax revenues with a low social cost.

Inelastic demand and supply.
Elastic demand and supply.

The two graphs illustrate the impact of the elasticity of demand and supply on the social cost of a tax and on the revenue generated by that tax.

In the graph on the left, demand and supply are inelastic, which means that the quantity demanded and offered does not change much in response to a change in price. When a tax is imposed, government revenue is represented by the large rectangle below the tax line and between the quantities before and after the tax. The social cost, which is the deadweight loss resulting from the tax, is relatively small as indicated by the small triangular area above the revenue rectangle and to the right of the tax line. This is due to the fact that the quantity traded decreases only slightly despite the tax, indicating that the transactions that no longer occur because of the tax are limited.

In the graph on the right, demand and supply are elastic, meaning that the quantity demanded and offered changes considerably in response to a change in price. Here, the tax leads to a greater reduction in the quantity traded, as indicated by the greater distance between the quantities before and after the tax. Government revenue is still represented by the rectangle below the tax line, but it is smaller because of the greater reduction in quantities traded. In addition, the social cost, or deadweight loss, is considerably greater, as shown by the large triangular area, because many more beneficial transactions do not occur because of the tax.

In short, these graphs show that goods with inelastic demand and supply are less sensitive to taxes and entail a lower social cost for a given level of income. Conversely, taxing goods with elastic demand and supply leads to a greater loss of economic efficiency and lower tax revenues for the government. This highlights the importance for decision-makers of understanding the elasticities of goods and services when designing tax systems to minimise the social cost while maximising the necessary tax revenues.

Review of the Lump Sum Tax and its Implications[modifier | modifier le wikicode]

The lump sum tax is often cited in economic theory as one of the most efficient forms of taxation from the point of view of resource allocation. One of the main characteristics of the lump sum tax is that it is independent of the economic behaviour of individuals: it is due regardless of the consumption, production or work carried out by a person. Because it does not alter the marginal incentives to work, invest or consume, it does not distort economic decisions and therefore does not lead to a loss of efficiency in the allocation of resources.

The advantages of simplicity and administrative efficiency are also significant. A flat tax eliminates the need for complex and costly taxation systems, reducing administrative costs for government and compliance costs for taxpayers.

However, the flat tax poses a major equity problem. It is regressive, meaning that all individuals pay the same absolute amount regardless of their ability to pay. This can result in a disproportionate burden on low-income individuals compared to high-income individuals, increasing inequality and potentially being seen as unfair. The poll tax introduced under Margaret Thatcher's government is a case in point: it was extremely unpopular, partly because it was perceived as unfair, and led to mass protests and its eventual repeal.

In practice, governments seek to balance efficiency and fairness in their tax systems. They often use a combination of different types of tax, including income taxes which are generally progressive, consumption taxes such as VAT, and sometimes flat-rate taxes for specific uses, to finance public spending while trying to maintain a degree of equity.

In the field of tax economics, one idea that is gaining in popularity is that of a moderately progressive or even proportional tax system, in order to reduce distortions in incentives to work and invest. Renowned economists such as Arthur Laffer, who popularised the famous "Laffer Curve" in his book "The Laffer Curve: Past, Present, and Future", point out how high tax rates can discourage extra effort, as much of the increase in income is absorbed by tax. The theory behind this is that when individuals receive a large proportion of their extra income lost through taxes, their motivation to work harder or take economic risks diminishes. Conversely, redistribution, rather than being achieved through a highly progressive tax structure, can be more effectively achieved through targeted government spending. This is partly because income transfers and social programmes can be targeted directly at less well-off households, without discouraging the productive activities of better-off individuals. For example, the implementation of assistance programmes such as the Supplemental Nutrition Assistance Program (SNAP) in the United States, better known as "food stamps", aims to provide food assistance to low-income families without directly impacting work incentives.

In addition, a simplified tax system reduces administrative costs and can reduce tax evasion and avoidance. Tax simplicity promotes understanding and compliance, as demonstrated by New Zealand's tax reform of the 1980s, which significantly simplified the personal and corporate income tax system, as described by Michael Cullen in "The New Zealand Tax Reform - A History". However, the economic stimulus expected from less progressive taxation must be balanced against the need for social equity. Authors such as Thomas Piketty in "Capital in the 21st Century" have highlighted the growing risks of inequality associated with less progressive tax systems. Although economic growth can be promoted by leaving more income available for consumption and investment, there is an ongoing debate about the level of progressivity needed to maintain a fair society.

The consensus that seems to be emerging among economists is not absolute, however. Tax policy choices depend very much on the specific socio-economic context of each country. In his book "Public Economics", Joseph Stiglitz offers an in-depth examination of how governments can structure tax systems to achieve both efficiency and equity, stressing that targeted government spending is often preferable to redistribution via taxation. Ultimately, the balance between economic efficiency and equity remains a complex policy dilemma, with profound implications for social cohesion and economic prosperity.

Various Tax Applications and Their Consequences[modifier | modifier le wikicode]

The concept of consumer and producer surplus is a fundamental tool in economics for assessing how different policies affect welfare in a market. It allows us to analyse the effects of government interventions such as taxes, minimum prices and maximum prices, and to understand the redistribution of economic surplus as well as the emergence of deadweight losses.

Let's start with the example of a minimum price, such as a government-imposed minimum wage. On a graph of labour supply and demand, the minimum wage is represented by a horizontal line above the market equilibrium point. The immediate effect is that the real wage is raised above the equilibrium level, which can reduce the demand for labour by employers while increasing the supply of labour by employees. This creates a surplus of labour, commonly known as unemployment. The surplus for consumers, in this case workers, increases for those who remain employed because they receive a higher wage. However, producers, or employers, suffer a loss of surplus because they have to pay more for the same amount of work. The deadweight loss is the loss of employment transactions that would have taken place without the price floor and represents the cost to the community in terms of increased unemployment and less economic output.

With regard to a maximum price, such as rent control, the government sets a ceiling price for rents which is generally below the market equilibrium point. This is supposed to make housing more affordable for tenants. On a graph of housing supply and demand, the maximum price is represented by a horizontal line below the market equilibrium point. The lower price increases the quantity of housing demanded while reducing the quantity offered, creating a shortage. Tenants benefit from an increased surplus because they pay less for housing than they would have been prepared to pay. However, landlords, or housing producers, lose part of their surplus because they receive less income from renting. The deadweight loss in this case represents the welfare lost as a result of dwellings not being built or rented, which would have been available without price controls.

To sum up, the introduction of a minimum or maximum price leads to a redistribution of economic surplus and a loss of efficiency that manifests itself as a deadweight loss. While such interventions may be motivated by equity or social policy considerations, they may also lead to unintended side effects that need to be carefully weighed by policy makers.

Summary and outlook[modifier | modifier le wikicode]

Consumer and producer surplus are central concepts in economics for assessing the well-being of market participants. Consumer surplus is graphically represented by the area below the demand curve and above the price level. This measures the benefit that consumers derive from purchasing goods and services, over and above what they actually pay. Producer surplus, on the other hand, is the area above the supply curve and below the price level, indicating the profit that producers make from selling their goods and services, over and above their production costs.

Market efficiency is measured by the maximisation of total surplus, which is the sum of consumer and producer surplus. In an ideal market, the 'invisible hand' of the market, a term popularised by Adam Smith in his book 'The Wealth of Nations', would act to allocate resources in such a way as to maximise this total surplus, making government intervention unnecessary.

However, real markets are often imperfect and subject to various types of failure, such as externalities, asymmetric information or monopolies, which can prevent efficiency from being achieved. In these cases, government intervention, in the form of regulation or taxation, can potentially lead to a more efficient allocation of resources.

The introduction of a tax has the effect of reducing both consumer and producer surplus, often more significantly than the revenue it generates for the government, resulting in a deadweight loss for society. This deadweight loss represents the social cost of the tax, symbolising the beneficial transactions that do not occur because of the tax burden.

Taxes tend to have a lower social cost in cases where they are applied to markets characterised by inelastic demand and/or supply. In other words, when consumers or producers do not react strongly to price changes, taxes can generate significant government revenues without causing an excessive reduction in market transactions.

It is therefore crucial to design tax systems that take these principles into account to ensure both efficiency and fairness, while providing the revenues needed to finance public goods and services. Economists such as Arthur Pigou, in his work "The Economics of Welfare", have explored these ideas, emphasising the importance of aligning tax interventions with broader economic and social policy objectives.

Annexes[modifier | modifier le wikicode]

References[modifier | modifier le wikicode]

[1] Page personnelle de Federica Sbergami sur le site de l'Université de Genève

[2] Page personnelle de Federica Sbergami sur le site de l'Université de Neuchâtel

[3] Page personnelle de Federica Sbergami sur Research Gate

[1]

[2]

[3]